
The moment of inertia calculation for a uniform rod involves expressing any mass element in terms of a distanceelement dr along the rod. When the mass element dm is expressed in terms of a length element dr along the rod and the sum taken over the entire length, the integral takes the form: The general form for the moment of inertia is: The resulting infinite sum is called an integral. The moment of inertia of a point mass is given by I = mr 2, but the rod would have to be considered to be an infinite number of point masses, and each must be multiplied by the square of its distance from the axis. If the thickness is not negligible, then the expression for I of a cylinder about its end can be used.Ĭalculating the moment of inertia of a rod about its center of mass is a good example of the need for calculus to deal with the properties of continuous mass distributions. The moment of inertia about the end of the rod is The moment of inertia about the end of the rod can be calculated directly or obtained from the center of mass expression by use of the Parallel axis theorem. HyperPhysics***** Mechanics ***** Rotationįor a uniform rod with negligible thickness, the moment of inertia about its center of mass is This process leads to the expression for the moment of inertia of a point mass. This provides a setting for comparing linear and rotational quantities for the same system.
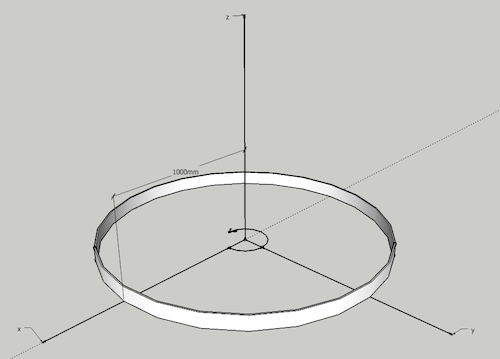
If the mass is released from a horizontal orientation, it can be described either in terms of force and accleration with Newton's second law for linear motion, or as a pure rotation about the axis with Newton's second law for rotation.
Moment of Inertia Rotational and Linear ExampleĪ mass m is placed on a rod of length r and negligible mass, and constrained to rotate about a fixed axis.


 0 kommentar(er)
0 kommentar(er)
